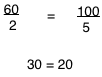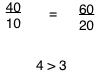Econowaugh AP: 11/23/16

Least-Cost Rule

The Resource Costs Cheat Sheet is here.

Least Cost Rule: production at least cost requires the ratio of labor’s marginal product to its price equals the ratio of capital’s marginal product to its price. The amounts of labor and capital employed must be adjusted, all the while keeping output constant, until this condition is achieved.

Simple, yes. (NO) The least cost rule comes into affect when the college board asks us to evaluate two inputs for production. Labor and Capital,,, workers and machines.

We are usually given two inputs, (labor and capital) and asked to evaluate which we need to buy more.

First - Let's create our own simple problem.

The price of labor is $10 and the price (rent) on capital is $20. The marginal product of labor is 40 and the marginal product of capital is 60. Should we hire more labor or more capital?

Remember the formula:

Set up the numbers:

What does this mean:

The marginal product of the last input of labor was 40 units produced and that labourer was paid $10. So for each $1 spent we received 4 units produced. 40/10 = 4

The marginal product of the last input of capital was 60 units produced and the rent was $20. So for each $1 spent we received 3 units produced. 60/20 = 3

Answer - Obviously we would want to hire more labor as (per dollar spent) on labourers produce a higher level of output. We want the biggest bang for the buck.

This is the simplest most straight forward way of presenting these problems don't expect it.

The legend of John Henry stands strong in American Folklore. Legend has it that Henry’s prowess as a steel driver was measured in a race against the new steam powered hammers being used to drill into rock to make holes for explosives to blast tunnels for the railroad back in the late 1800’s. In a battle of man versus machine, Henry outdueled the steam powered technology, only to die in victory with his hammer in his hands as his heart gave out from the stress. Where it actually happened, or if it even happened at all, will always be in debate but the legend will always be a firm reminder of how technology finds itself taking over tasks previously done by hand.

(2012 AP Multiple Choice)

Answer - (A) The marginal product per dollar spent on labor is equal to the marginal product per dollar spent on capital.

2000 AP Multiple Choice Question

To answer this question you must understand that the Profit Max rule is about setting the ratios equal to one. There is a point where the right combination of labor and capital is maximised, this is always at the profit max (MR=MC) point on our graphs. If we are at Profit Max then the least cost rule is attained/satisfied.

Lets plug in some numbers: for answer (A)

This combination of capital and labor we would want to hire more labor.

Lets plug in the numbers for (E)

Answer (E) Both sides (ratios) equal each other. We are at profit max = least cost combination.

2008 AP Multiple Choice Question

Formula:

OK, so we can see clearly that we would want to hire more (increase) labor as the output per dollar is greater for labor. But, the confusion is should we choose answer (D) or (E).

The marginal product of the last input of labor was 40 units produced and that labourer was paid $10. So for each $1 spent we received 4 units produced. 40/10 = 4

If we increase the amount of labor we hire the MP will fall due to diminishing marginal returns.

The marginal product of the last input of capital was 60 units produced and the rent was $20. So for each $1 spent we received 3 units produced. 60/20 = 3

If we decrease the amount of capital we hire the MP will rise due to increasing marginal returns.

Remember, it is the least-cost rule, we want the least inputs we can hire(rent) to get to profit max.

Answer - (E) Increase labor and decrease capital.

(Practise Problem)